top
introduction
directions
Benchmark Problems
Papers
 
Benchmark Problem B0-1F

-Properties-
category
type
index
domain
object
note
B. interior
0. basic
B0-1F
frequency
cubic cavity
scattering

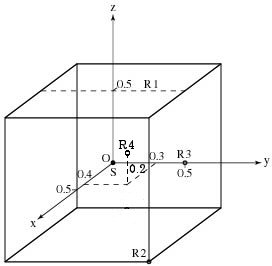Geometry:
Cubic cavity (1 m * 1 m * 1 m)

Boundary condition:
All faces are rigid boundaries. A point source (S) is located at (0, 0, 0), assuming its stationary vibration with volume acceleration amplitude 1.0 m3/s2.
Fig. B0-1F-1: Geometry of problem B0-1F (unit: m).
-Task A-

Distribution:
Sound pressure amplitude [Pa] at 101 points (R1), on the line x = 0, z = 0.5, located at 0.01 m intervals (y = -0.5, ..., 0.5). At the octave-band mid-frequencies (f = 31.5, 63, 125, 250, 500, 1k, 2k, 4k Hz).

Dataform:
d-b0-1fa.xls

Contribution:

  • [06/03/13] B0-1FA-07: FEM, Yamamura, K.
  • [05/03/08] B0-1FA-06: Fast Multipole BEM, Yasuda, Y.
  • [02/06/04] B0-1FA-05: BEM (SYSNOISE), Strauch, O.
  • [02/05/29] B0-1FA-04: Fast Multipole BEM, Yasuda, Y.
  • [02/05/24] B0-1FA-03: BEM, Yasuda, Y.
  • [02/04/25] B0-1FA-02: FEM (SoundSolve, ITA TU Aachen), Franck, A.
  • [02/04/23] B0-1FA-01: Mode summation, Svensson, P.
Ref. comparison
-Task B-


Frequency response:
Sound pressure amplitude [Pa] at 3 points (0.5, 0.5, -0.5) (R2), (0, 0, 0.5) (R3) and (0.4, 0.3, 0.2) (R4). At the frequencies with 1 Hz intervals from 20 Hz to 4 kHz, or with 10 Hz intervals from 20 Hz to 4 kHz.

Dataform:
d-b0-1fb.xls

Contribution:
  • [06/03/13] B0-1FB-07:FEM, Yamamura, K.
  • [03/10/15] B0-1FB-06:Fast Multipole BEM (Improved), Oshima, T.
  • [02/06/04] B0-1FB-05: BEM (SYSNOISE), Strauch, O.
  • [02/05/29] B0-1FB-04: Fast Multipole BEM, Yasuda, Y.
  • [02/05/24] B0-1FB-03: BEM, Yasuda, Y.
  • [02/04/25] B0-1FB-02: FEM (SoundSolve, ITA TU Aachen), Franck, A.
  • [02/04/23] B0-1FB-01: Mode summation, Svensson, P.
Ref. comparison
-Reference-

Visualization of results:
  • FEM, Yamamura, K.
    		•